Canonical projection of a lattice in
is based upon the idea of decomposing in terms of orthogonal spaces E and 
and projecting select portions of the lattice in onto E. In particular, some compact set, C, in is chosen. The "canonical" choice for C is the projection of Voronoi cell of the origin in , relative to the lattice, as projected onto . The Voronoi cell around a point in a collection of points is the set of points in space that are at least as close to the given point as any other point in the collection. We also shift (translate) the points before we project and test them; one can equivalently describe the compact set C as shifted, but for convenience, we do our shift in . The points which are projected onto E are the points of the lattice which map to C under shift and projection to . In other words, the preimage of C corresponds to a cylinder in , compact in some directions and unbounded in others. The points of the lattice in which are in that cylinder are projected onto E.
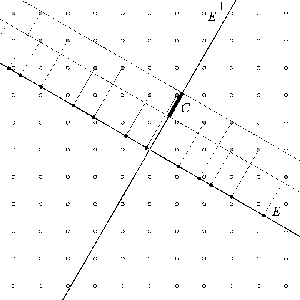
The figure illustrates canonical projection in a simpler situation than we use, but it serves as a useful overview of the process. In the figure, the orthogonal spaces E and are the marked lines and together they generate . A compact set C in is marked in black. The set C defines a cylinder in which lies between the dashed lines. Some of the integer lattice points lie in the cylinder—these are marked as points with gray centers. Others lattice points lie outside the cylinder—these are marked as points with hollow centers. Those that are in the cylinder are projected onto E along dotted lines to points marked with black circles. The result of Canonical projection of the lattice is that point set in E.
The projections we use are not as simple. We are interested in the case when E is 2-dimensional and seek to connect the projected points with edges to give a tiling. Moreover, the compact sets we use tend to be quite complex. Our example on E8 involves a polytope with more than 40,000 facets as the compact set C.