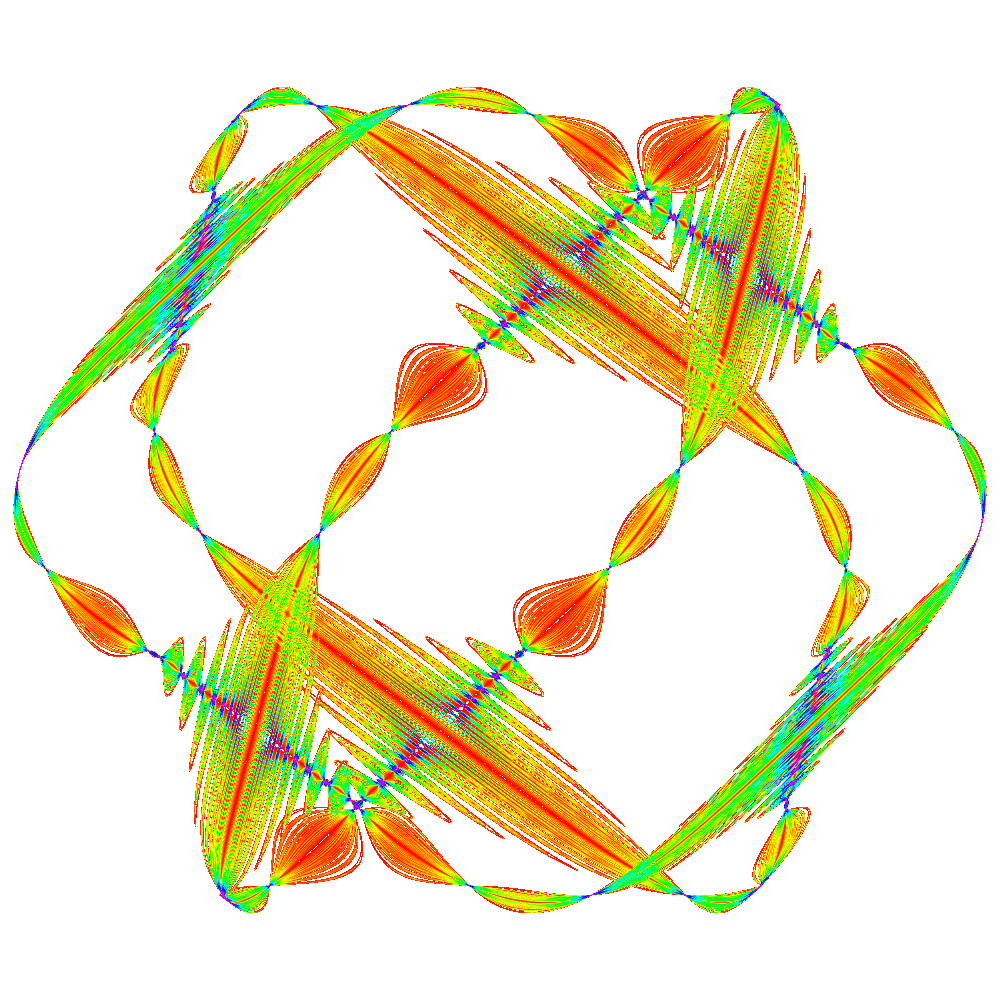
This image is of a chaotic attractor that has the symmetry of a tetrahedron. This figure appears in:
Clifford A. Reiter, "Chaotic Attractors with the Symmetry of the Tetrahedron", Computers & Graphics, 21 6 (1997) 841-848.
Notice a tetrahedron sits nicely inside a cube (take alternate vertices) and hence the symmetry
of the tetrahedron is related to that for the cube. In fact, you might imagine a cube sitting inside
this attractor, but notice fourth turns of the cube would wreck the orientation of the attractor. Thus,
this attractor does not have the full symmetry of the cube. Attractors with the symmetry of the cube
were investigated by an REU group in 1995 and their results have appeared in:
Gabriel F. Brisson, Kaj M. Gartz, Benton J. McCune, Kevin
P. O'Brien, and Clifford A. Reiter, "Symmetric Attractors in
Three-dimensional Space", Chaos, Solitons & Fractals, 7 7 (1996)
1033-51.
Back to Cliff's Home Page
Back to Miscellaneous Fractals Gallery

